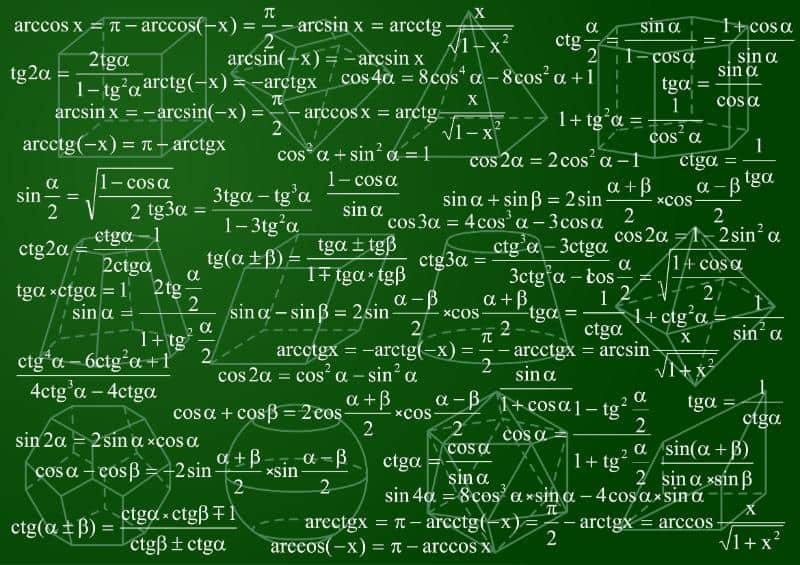
Headings for doing math homework.
Mathematics is a subject that requires a structured approach and clear headings to ensure that the concepts are well understood and the problems are solved correctly. Here are some helpful headings for doing math homework:
(Looking for a “Math pro specialist“? Contact us today!)

Introduction to Mathematical Proofs: Understanding the Basics
Mathematical proofs are a fundamental aspect of advanced mathematics, and they play a crucial role in establishing the validity of mathematical statements. Proofs provide logical arguments and evidence to support mathematical claims, ensuring that mathematical results are reliable and accurate. Understanding the basics of mathematical proofs is essential for students pursuing higher-level math courses or careers in fields that require rigorous logical reasoning. Here are some key concepts and strategies to consider when engaging with mathematical proofs.
Logical Structure: A mathematical proof follows a logical structure that consists of a series of statements and their justifications. Each statement builds upon the previous ones, leading to the desired conclusion. It is essential to present the proof in a clear, organized manner, highlighting the logical connections between statements.
Assumptions and Axioms: Mathematical proofs are based on a set of assumptions and axioms, which are self-evident truths or established mathematical principles. These assumptions and axioms serve as the foundation for logical reasoning and are accepted as true within the context of the proof. It is important to clearly state any assumptions or axioms used in the proof to ensure transparency and clarity.
Proof Techniques: Various proof techniques can be employed to establish the validity of a mathematical statement. These techniques include direct proofs, indirect proofs (proofs by contradiction), proof by contrapositive, proof by induction, and proof by cases. Each technique has its own approach and requires careful reasoning and logical deductions. Understanding these techniques and when to apply them is essential for constructing effective proofs.
Definitions and Terminology: Mathematical proofs often involve the use of specialized terminology and definitions. It is important to clearly define any terms or symbols used in the proof to avoid ambiguity and misunderstandings. Precise language and consistent notation contribute to the clarity and coherence of the proof.
Logical Reasoning: Logical reasoning is at the heart of mathematical proofs. It involves making logical deductions based on the information provided and the rules of logic. The use of logical connectives such as “if-then” statements, conjunctions, disjunctions, and negations helps establish the logical flow of the proof. Paying attention to the logical connections between statements and ensuring that each step is justified based on previously established facts is crucial for constructing a valid proof.
Counterexamples: Counterexamples can be valuable tools in mathematical proofs. By providing specific examples that refute a given statement, counterexamples help identify the conditions under which a statement fails to hold true. Including counterexamples in a proof strengthens its validity by showing that the statement is not true in all cases.
Proof Practice: Engaging in regular practice with mathematical proofs is essential for developing proficiency in constructing and understanding them. Solving proof-based exercises and working through examples can help build intuition, enhance logical reasoning skills, and familiarize students with common proof techniques.
Collaboration and Resources: Mathematics is often best learned through collaboration and access to helpful resources. Engaging in discussions with peers, seeking guidance from teachers or tutors, and utilizing textbooks or online materials dedicated to proof theory can provide valuable insights and support for understanding and constructing proofs effectively.
Mathematical proofs are a fundamental aspect of mathematics that contribute to its rigor, precision, and reliability. By understanding the basics of proof theory, students can enhance their problem-solving abilities, develop critical thinking skills, and gain a deeper appreciation for the logical foundations of mathematics. Through practice, patience, and a systematic approach, students can become proficient in constructing and understanding mathematical proofs, opening doors to further exploration and discovery in the vast realm of mathematics.