What terms in mathematics are comparable?
The New York State Next Generation Mathematics Learning Standards include comparisons in a variety of contexts, from counting, adding and subtracting for young students to percentages, fractions, rates and ratios, proportional thinking and probability for older students. Regardless of the type of comparison used, there is one thing all students need to know: they can only use a mathematical strategy if they understand it and can explain how they came up with the solution to a problem in a logical way that is clear to their audience.
(Looking for”delta math answers“? Contact us Today!)
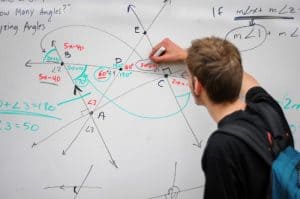
Identify the most important comparison in mathematics (the one that actually works) by finding an answer that is both correct and reasonable. This requires understanding and implementing mathematical strategies in a way that reflects the standard’s content and is relevant to the students’ needs and interests.
Determine the most impressive and most useful comparison in mathematics involving a number of objects, symbols and/or processes that is mathematically accurate and applicable to real-world problems. This includes a formal model, a visual or verbal model, or any other method that clearly communicates the steps necessary to achieve the desired result.
The most impressive comparison is the one that is the smallest relative to a number of objects, symbols or processes. It also has to do with the smallest quantity of something that is mathematically significant, e.g., the smallest number that is a positive integer. The smallest number is the best of both worlds because it contains all the information about a mathematical concept while also displaying the biggest bang for your buck.

